Complete Solution with Step Input

We next consider the complete solution (composed of both homogeneous and particular solutions) of the 2nd order DE

$$y''(t)+2\zeta\omega_n y'(t)+\omega_n^2 y(t)=x(t)$$ (232)

with a unit step $x(t)=u(t)$ input and zero initial conditions $y(0)=\dot{y}(0)=0$. As the input is a constant for $t>0$, we can assume the particular solution to be a constant $y_p(t)=C$ with zero derivatives $y'_p(t)=y''_p(t)=0$. Substituting these into the DE above, we get $y_p(t)=C=1/\omega_n^2$, i.e., the steady state solution is:

$$y_{ss}(t)=y_p(t)=y(\infty)=\frac{1}{\omega_n^2}$$ (233)

The complete response can be obtained as the sum of the homogeneous solution (same as that obtained previously) and particular solution, corresponding to the transient and steady state response, respectively:

$$y(t)=y_h(t)+y_p(t)=C_1 e^{s_1t}+C_2 e^{s_2t}+\frac{1}{\omega_n^2}$$ (234)

The two coefficients $C_1$ and $C_2$ can be obtained based on the two zero initial conditions:

$$y(0) = y(t)\bigg\vert _{t=0}=C_1+C_2+\frac{1}{\omega_n^2}=0$$

$$\dot{y}(0) = \dot{y}(t)\bigg\vert _{t=0}=C_1s_1+C_2s_2=0$$ (235)

Solving these equations we get:

$$C_1=\frac{s_2}{\omega_n^2(s_1-s_2)}=\frac{-s_2}{\omega_n^2(s_2-s_1)}, \;\;\;\;\;\;C_2=\frac{s_1}{\omega_n^2(s_2-s_1)}$$ (236)

Now the complete solution becomes:

$$y(t)=\frac{1}{\omega_n^2}\left[1-\left(\frac{s_2e^{s_1t}}{s_2-s_1... ...ht] =\frac{1}{\omega_n^2}\left(1-\frac{s_2e^{s_1t}-s_1e^{s_2t}}{s_2-s_1}\right)$$ (237)

Alternatively, the nonhomogeneous 2nd-order LCCODE given above can be converted into a 1st-order ODE system and solving which we obtain the same results, as shown here.

The two roots $s_1$ and $s_2$ take different forms depending on whether the discriminant $\Delta=4\omega_n^2(\zeta^2-1)$ is greater or smaller than 0, i.e., whether $\zeta$ is greater or smaller then 1. Here we only consider the case when $0 < \zeta < 1$, i.e., $\Delta<0$, for an under-damped second order system. The two roots are

$$s_{1,2}=\omega_n \; (-\zeta\pm j\sqrt{1-\zeta^2})=-\zeta \omega_n\pm j\omega_d, \;\;\;\; \;\;\;\;s_2-s_1=-2j\omega_d$$ (238)

where $\omega_d$ is the damped natural frequency:

$$\omega_d=\omega_n\sqrt{1-\zeta^2}$$ (239)

Finally the complete solution of the non-homogeneous DE is:

$$y(t) = \frac{1}{\omega_n^2}\left[1-\left(\frac{s_2}{s_2-s_1}e^{s_1t}-\frac{s_1}{s_2-s_1}e^{s_2t}\right)\right]$$

$$= \frac{1}{\omega_n^2}\left[ 1- \left(\frac{\zeta+j\sqrt{1-\zeta^2}... ...1-\zeta^2}}{2j\sqrt{1-\zeta^2}} e^{(-\zeta\omega_n-j\omega_d)t} \right) \right]$$

$$= \frac{1}{\omega_n^2}\left[ 1-\frac{e^{-\zeta\omega_nt}}{\sqrt{1-\... ...j\omega_dt} -\frac{\zeta-j\sqrt{1-\zeta^2}}{2j} e^{-j\omega_dt} \right) \right]$$

$$= \frac{1}{\omega_n^2}\left[ 1-\frac{e^{-\zeta\omega_nt}}{\sqrt{1-\... ...rac{ e^{j\phi} e^{ j\omega_dt}-e^{-j\phi} e^{-j\omega_dt} }{2j} \right) \right]$$

$$= \frac{1}{\omega_n^2}\left[1-\frac{e^{-\zeta\omega_nt}}{\sqrt{1-\zeta^2}} \sin(\omega_dt+\phi) \right]$$ (240)

where

$$\phi=\tan^{-1}\left( \frac{\sqrt{1-\zeta^2}}{\zeta} \right), \;\;\;\; \;\;\;\; \sin \phi=\sqrt{1-\zeta^2},\;\;\;\;\cos \phi=\zeta$$ (241)

The step response $y(t)$ can be characterized by two parameters:

• Rise time:

  The rise time $t_r$ is the time at which $y(t)$ reaches $100\%$ of the steady state value $y(t_r)=1$ (assumed to be 1 for simplicity):

  $$1-\frac{e^{-\zeta\omega_nt_r}}{\sqrt{1-\zeta^2}} \sin(\omega_dt_r+\phi)=1 \;\;\;\;\; \;\;\;\;\; \frac{e^{-\zeta\omega_nt_r}}{\sqrt{1-\zeta^2}} \sin(\omega_dt_r+\phi)=0$$ (242)

  i.e.,

  $$\sin(\omega_dt_r+\phi)=0 \;\;\;\;\; \;\;\;\;\; \omega_dt_r+\phi=\pi$$ (243)

  Solving for $t_r$ we get

  $$t_r=\frac{\pi-\phi}{\omega_d}=\frac{\pi-\phi}{\omega_n\sqrt{1-\zeta^2}}$$ (244)

  But as $\tan(\pi-\phi)=-\tan\phi=-\sqrt{1-\zeta^2}/\zeta$, taking arctangent on both sides we get

  $$\pi-\phi=\tan^{-1}\left(-\frac{\sqrt{1-\zeta^2}}{\zeta}\right), \;\;\;\;\; \;\;\;\;\; t_r=\frac{\pi-\phi}{\omega_d} =\frac{1}{\omega_n\sqrt{1-\zeta^2}} \tan^{-1}\left(-\frac{\sqrt{1-\zeta^2}}{\zeta}\right)$$ (245)

• Peak time:

  The peak time $t_p$ is the time at which $y(t)$ reaches the first peak, which can be found by setting the time derivative of $y(t)$ to zero, yielding

  $$\zeta\omega_ne^{-\zeta\omega_nt} \sin(\omega_dt+\phi) =e^{-\zeta\omega_nt} \omega_d\cos(\omega_dt+\phi)$$ (246)

  i.e.,

  $$\zeta\omega_n \sin(\omega_dt+\phi)=\omega_n\sqrt{1-\zeta^2}\cos(\omega_dt+\phi)$$ (247)

  or

  $$\tan(\omega_dt+\phi)=\frac{\sqrt{1-\zeta^2}}{\zeta}=\tan\phi$$ (248)

  We get $\omega_dt=\pi$ for the first peak, i.e.,

  $$t_p=\frac{\pi}{\omega_d}=\frac{\pi}{\omega_n\sqrt{1-\zeta^2}}$$ (249)

  Substituting $t_p$ inito $y(t)$ we get the peak value:
  

  $$y(t_p) = 1-\frac{e^{\zeta\pi/\sqrt{1-\zeta^2}}}{\sqrt{1-\zeta^2}} \sin(\pi+\phi)$$

  $$= 1+\frac{e^{\zeta\pi/\sqrt{1-\zeta^2}}}{\sqrt{1-\zeta^2}} \sqrt{1-\zeta^2} =1+e^{-\zeta\pi/\sqrt{1-\zeta^2}}$$ (250)

  
  The overshoot is $e^{-\zeta\pi/\sqrt{1-\zeta^2}}$.

In particular, if $R=0$ and therefore $\zeta=0$, we have

$$y(t)=\frac{1}{\omega_n^2}\left[1-\sin(\omega_n t+\pi/2)\right] =\frac{1}{\omega_n^2}\left[1-\cos(\omega_n t)\right]$$ (251)

The plots below shows an example with $f_n=1, \omega_n=2\pi$. Note the critical damped case when $\zeta=1$. An overshoot will occur for any $\zeta<1$.

The step response is plotted below. Note that $y(0)=0$ and $\dot{y}(0)=0$.

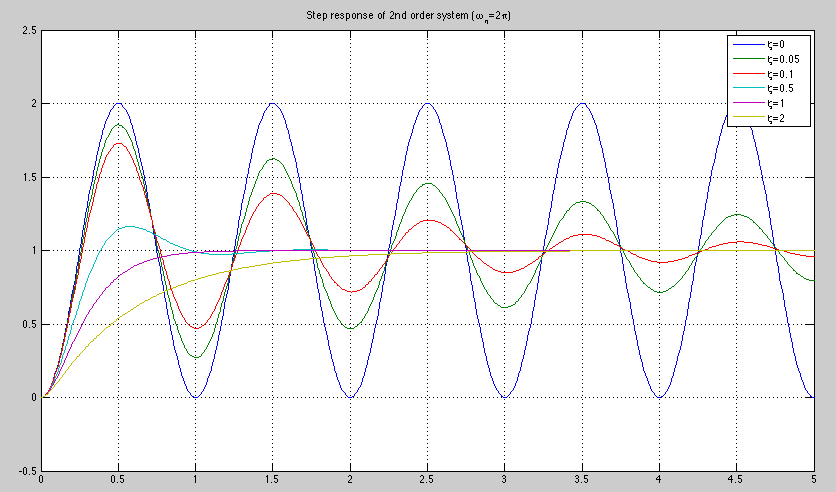
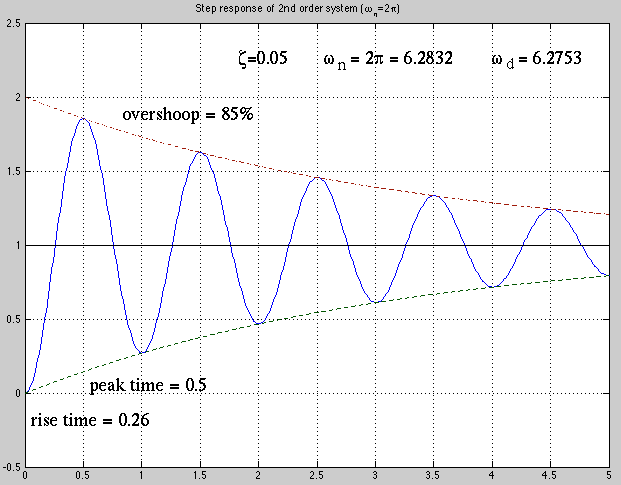
Example

Consider the response $y(t)=v_C(t)$ of an undamped 2nd order RCL system.

• Find the response $y(t)$ of the RLC circuit to a step input $x(t)=Vu(t)$. The general solution is the sum of the homogeneous solution $A\sin\omega_nt+B\cos\omega_nt$ and the particular solution $V$:

  $$y(t)=V+A\sin(\omega_nt)+B\cos(\omega_nt),\;\;\;\;\;\;\;t>0$$ (252)

  Consider two different sets of initial conditions:
  • Zero initial conditions $y(0)=\dot{y}(0)=0$. We have $y(0)=V+B=0$ and $\dot{y}(0)=A\omega_n=0$, and

    $$y(t)=V+A\sin(\omega_nt)+B\cos(\omega_nt)=V[1-\cos(\omega_nt)]$$ (253)

  • $y(0)=V$, $\dot{y}=0$. We have $y(0)=V+B=V$ and $\dot{y}(0)=A\omega_n=0$, i.e., $A=B=0$ and

    $$y(t)=V+A\sin(\omega_nt)+B\cos(\omega_nt)=V$$ (254)

• Find the system's response to a square impulse

  $$x(t)=\left\{ \begin{array}{ll}1 & 0\le t< t_0 \\ 0 & \mbox{else} \end{array} \right.$$ (255)

  As $x(t)=u(t)-u(t-t_0)$, the response is

  $$y(t)=[1-\cos(\omega_nt)]u(t)-[1-\cos(\omega_n(t-t_0))]u(t-t_0)$$ (256)

  When $t>t_0$, we have

  $$y(t)=\cos(\omega_n(t-t_0))-\cos(\omega_nt)$$ (257)

  We further consider two special cases.
  • When $t_0=T=2\pi/\omega_n$, we have
    

    $$y(t) = [1-\cos(\omega_nt)]u(t)-[1-\cos(\omega_n(t-T))]u(t-T)$$

    $$= \left\{\begin{array}{cl} 1-\cos(\omega_nt) & 0<t<T \\ 0 & \mbox{else} \end{array} \right.$$ (258)

    
    This is a one period of a sinusoid.
  • When $t_0=T/2=\pi/\omega_n$, we have
    

    $$y(t) = [1-\cos(\omega_nt)]u(t)-[1-\cos(\omega_n(t-T/2))]u(t-T/2)$$

    $$= [1-\cos(\omega_nt)]u(t)-[1+\cos(\omega_nt)]u(t-T/2)$$

    $$= \left\{\begin{array}{cl}1-\cos(\omega_nt) & 0<t<T/2 \\ -2\cos(\omega_nt) & t>T/2 \end{array} \right.$$ (259)

    
    This is a pure sinusoid after $t>T/2$.

• Find the impulse response $h(t)$. The input $x(t)$ is an impulse which can be written as

  $$x(t)=\delta(t)=\lim_{t_0\rightarrow 0}\left\{ \begin{array}{cl} 1/t_0 & 0\le t< t_0 \\ 0 & \mbox{else} \end{array}\right.$$ (260)

  When $t_0\rightarrow 0$, we have first order approximations $\cos(\omega_nt_0)\approx 1$ and $\sin(\omega_nt_0)\approx \omega_nt_0$, and we get

  $$\cos(\omega_n(t-t_0))=\cos(\omega_nt)\;\cos(\omega_nt_0)+\sin(\omega_nt)\; \sin(\omega_nt_0) \approx\cos(\omega_nt)+\sin(\omega_nt)\,\omega_nt_0$$ (261)

  Substituting this into $y(t)=\cos(\omega_n(t-t_0))-\cos(\omega_nt)$ we get the impulse response

  $$y(t)=\omega_n\sin(\omega_nt)$$ (262)

• It is often desirable for a second order system to reach a set steady state value without overshoot. This can be achieved by driving the system by an impulse $\delta(t)/\omega_n$ with response $\sin(\omega_nt)$, followed by a step $u(t-T/4)$ with response

  $$[1-\cos(\omega_n(t-T/4))]u(t-T/4)=[1-\cos(\omega_nt-\pi/2)]u(t-T/4) =[1-\sin(\omega_nt)]u(t-T/4)$$ (263)

  The total response is

  $$y(t)=\sin(\omega_nt)u(t)+[1-\sin(\omega_nt)]u(t-T/4) =\left\{\begin{array}{cl} \sin(\omega_nt) & 0<t<T/4 \\ 1 & t>T/4\end{array} \right.$$ (264)

  We see that after $y(t)$ reaches the first peak of $1$ at $t=T/4$, it will stay at the constant value $1$ as the two responses cancel each other for $t>T/4$.

• Alternatively, the steady state value $v=1$ can be achieved without overshoot by applying an input $x(t)=[u(t)-u(t-T/2)]/2$. The response to $u(t)/2$ is

  $$\frac{1}{2}[1-\cos(\omega_nt)]u(t)$$ (265)

  while the response to $u(t-T/2)/2$ is

  $$\frac{1}{2}[1-\cos(\omega_n(t-T/2))]u(t-T/2) =\frac{1}{2}[1-\cos(\omega_nt-\pi)]u(t-T/2) =\frac{1}{2}[1+\cos(\omega_nt)]u(t-T/2)$$ (266)

  The overall response is the difference between the two individual responses:

  $$y(t)=\left\{\begin{array}{cl} [1-\cos\omega_nt]/2 & 0<t<T/2 \\ 1 & t>T/2 \end{array} \right.$$ (267)

  i.e., the response is $y(t)=1$ for all $t>T/2$.

• It is desirable for a second order system to reach a steady state value $y(t)=1$ within a time delay $t_0$ without overshoot. We first consider driving the system with a positive square pulse of value $V>0$ followed by a negative one of $(1-a)V<0$:

  $$x(t)=\left\{\begin{array}{cl} V & (0<t<t_0/2) \\ (1-a)V & (t_0/2<t<t_0) \end{array}\right. =V \left[u(t)-a u(t-t_0/2)\right]$$ (268)

  The response for $t_0/2<t<t_0$ is:

  $$y(t)=V\left[ 1-\cos(\omega_nt)-a[1-\cos(\omega_n(t-t_0/2))]\right]$$ (269)

  In order for the output to be a constant $y(t)=1$ for $t>t_0$, we need to have the input $x(t)=1$ for $t>t_0$, and set the initial conditions at $t=t_0$ to be $y(t_0)=1$ and $\dot{y}(t_0)=0$. To do so, we let

  $$\frac{dy(t)}{dt}\bigg\vert _{t=t_0}=V\omega_n\left[ \sin(\omega_n... ...right]_{t=t_0} =V\omega_n\left[ \sin(\omega_nt_0)-a\sin(\omega_nt_0/2)\right]=0$$ (270)

  Based on the trigonometric identity $\sin(\omega_nt_0)=2\sin(\omega_nt_0/2)\cos(\omega_nt_0/2)$, the equation above can be written as

  $$2\cos(\omega_nt_0/2) =a$$ (271)

  Substituting this into the desired initial condition $y(t_0)=1$, we get

  $$y(t_0)=V\left[ 1-\cos(\omega_nt_0)-2\cos(\omega_nt_0/2) [1-\cos(\omega_n(t_0/2))]\right] =2V(1-\cos(\omega_nt_0/2) =4V\sin^2(\omega_nt_0/4)=1$$ (272)

  where we have used the trigonometric identities $2\cos^2(\omega_nt_0/2)=1+\cos(\omega_nt_0)$ and $2\sin^2(\omega_nt_0/4)=1-\cos(\omega_nt_0/2)$. Solving this for $V$ we get

  $$V=\frac{1}{4\sin^2(\omega_nt_0/4)}$$ (273)

  As now we have the initial conditions $y(t_0)=1$ and $\dot{y}(t_0)=0$ as needed. If we set $x(t)=1$ for $t>t_0$, so that the output will be at constant $y(t)=1$ when $t>t_0$.