Impedance and Generalized Ohm's Law

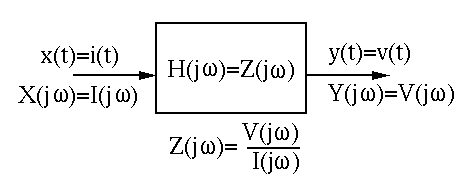
Impedance of Basic Components

In time domain, the relationship between the sinusoidal current through and the sinusoidal voltage across a capacitor or an inductor is described by a differential equation. However, in frequency domain, where these sinusoidal variables are represented as complex exponentials, and the components such as R, C, and L are all represented by their impedances, then the relationship between the sinusoidal voltage and current can be described by an algebraic equation.

Specifically, we represent the sinusoidal voltage and current as the projection of the corresponding vector in the complex plane rotating counter-clock wise onto the real axis:

$$v(t)=V_p\cos(\omega t+\phi)=Re[ V_p e^{j(\omega t+\phi)} ], \;\;\;\;\;\;\;\; i(t)=I_p\cos(\omega t+\psi)=Re[ I_p e^{j(\omega t+\psi)} ]$$ (33)

and define the impedance of a component (R, C, and L) as the ratio of the voltage and current associated with the component both in complex exponential form:

$$=Z=\frac{V_pe^{j(\omega t+\phi)}}{I_pe^{j(\omega t+\psi)}} =\frac... ...ac{\dot{V}}{\dot{I}} =\frac{\mbox{Phasor of voltage}}{\mbox{Phasor of current}}$$ (34)

This is the generalized version of the Ohm's law for AC circuits.

• Resistor:

  $$V_pe^{j(\omega t+\phi)}=R I_pe^{j(\omega t+\psi)}$$ (35)

  The impedance of a resistor is the ratio of the phasor representations of the voltage and current. As the current through and voltage across a resistor are always in phase, i.e., $\psi=\phi$, we have

  $$Z_R=\frac{V_p\,e^{j\phi}}{I_p\,e^{j\psi}} =\frac{V_p}{I_p}=R, \;\;\;\;\; \vert Z_R\vert=R,\;\;\;\;\;\angle Z_R=0$$ (36)

  The magnitude and phase of the current and voltage are related by:

  $$V_p=\vert Z_R\vert\,I_p=R\,I_p,\;\;\;\;\;\; \angle\dot{V}=\angle\dot{I}$$ (37)

  The resistor introduces no phase shift between the voltage and current, i.e., they are in phase.

• Capacitor:

  $$i(t)=I_pe^{j(\omega t+\psi)}=C\frac{dv}{dt} =C\frac{d}{dt}[V_p e^{j(\omega t+\phi)}] =j\omega C V_p e^{j(\omega t+\phi)}$$ (38)

  The impedance of a capacitor is the ratio of the phasor representations of the voltage and current:

  $$Z_C=\frac{V_pe^{j\phi}}{I_pe^{j\psi}}=\frac{1}{j\omega C}=\frac{-... ...\;\;\;\;\vert Z_C\vert=\frac{1}{\omega C},\;\;\;\;\;\;\angle Z_C=-\frac{\pi}{2}$$ (39)

  The magnitude and phase of the current and voltage are related by:

  $$\dot{V}=Z_C\dot{I}=-j\dot{I}/\omega C,\;\;\;\;\; V_p=I_p/\omega C,\;\;\;\;\;\; \angle\dot{V}=\angle\dot{I}-\frac{\pi}{2}$$ (40)

  The phase shift introduced by a capacitor is $\angle Z_C=-\pi/2=-90^\circ$, i.e., the voltage lags behind the current by $90^\circ$, or the current leads the voltage by $90^\circ$ (“ICE”).

• Inductor:

  $$v(t)=V_pe^{j(\omega t+\phi)}=L\frac{di}{dt} =L\frac{d}{dt}[I_p e^{j(\omega t+\psi)}] =j\omega L I_p e^{j(\omega t+\psi)}$$ (41)

  The impedance of an inductor is the ratio of the phasor representations of the voltage and current:

  $$Z_L =\frac{V_pe^{j\phi}}{I_pe^{j\psi}} =j\omega L, \;\;\;\;\vert Z_L\vert=\omega L,\;\;\;\angle Z_L=\frac{\pi}{2}$$ (42)

  The magnitude and phase of the current and voltage are related by:

  $$\dot{V}=Z_L\dot{I}=j\omega L\dot{I},\;\;\;\;\; V_p=\omega LI_p,\;\;\;\;\;\; \angle\dot{V}=\angle\dot{I}+\frac{\pi}{2}$$ (43)

  The phase shift introduced by a inductor is $\angle Z_L=\pi/2=90^\circ$, i.e., the voltage leads the current by $90^\circ$ (“ELI”).

One way to remember the phase between the voltage $E$ and current $I$ associated with capacitor $C$ and inductor $L$ is “ELI the ICE man”. Also, consider two extreme cases:

• When $\omega=0$, $Z_C\rightarrow \infty$ and the capacitor has zero conductivity due to the insulation between its two plates (open circuit), and $Z_L=0$ as there is no flux change in the inductor and the resistance of the coil is ideally zero.
• When $\omega\rightarrow \infty$, $Z_C\rightarrow 0$ and the capacitor becomes highly conductive, and $Z_L\rightarrow \infty$ as the self-induced voltage in the coil always acts against any change in the input (Lenz's Law).

In a DC circuit, each resistor is measured by either its resistance $R$ or its conductance $G=1/R$. In an AC circuit each component (capacitor, inductor, or resistor) is measured by its impedance $Z$, of which the real and imaginary parts are respectively the resistance $R$ and reactance $X$, or its admittance $Y=1/Z$, of which the real and imaginary parts are respectively the conductance $G$ and susceptance $B$, as summarized below:

• Impedance

  As a complex variable, the impedance $Z$ can be written in either Cartesian or polar form:

  $$Z=R+jX=\vert Z\vert e^{j\angle Z}=\vert Z\vert\angle Z$$ (44)

  • The real part of impedance $Re[Z]=R$ is called resistance.
  • The imaginary part of impedance $Im[Z]=X$ is called reactance.
  Impedance, resistance, and reactance are all measured by the same unit Ohm ($\Omega$).

  The magnitude and phase angle of $Z$ are:

  $$\left\{\begin{array}{l} \vert Z\vert=\sqrt{R^2+X^2}\\ \angle Z=\t... ...} R=\vert Z\vert\cos\angle Z\\ X=\vert Z\vert\sin\angle Z\\ \end{array}\right.$$ (45)

  The impedances $Z_C=1/j\omega C=-j/\omega C$ associated with $C$ and $Z_L=j\omega L$ are both purely imaginary, i.e., they are both reactance, indicating these components are reactive and consume no energy.

• Admittance

  The reciprocal of the impedance $Z$ is called admittance:

  $$Y=\frac{1}{Z}=\frac{1}{R+jX}=\frac{R-jX}{R^2+X^2} =\frac{R}{R^2+X^2}+j\frac{-X}{R^2+X^2}=G+jB$$ (46)

  • The real part of admittance is called conductance:

    $$G=Re[Y]=\frac{R}{R^2+X^2} \ne \frac{1}{R}$$ (47)

  • The imaginary part of admittance is called susceptance:

    $$B=Im[Y]=\frac{-X}{R^2+X^2} \ne \frac{1}{X}$$ (48)

  Admittance, conductance and susceptance are all measured by the same unit Siemens ($S$).

  The magnitude and phase of complex admittance are

  $$\vert Y\vert=\sqrt{G^2+B^2}=\frac{1}{\sqrt{R^2+X^2}},\;\;\;\;\; \... ...an^{-1} \left(\frac{B}{G}\right)=\tan^{-1} \left(\frac{-X}{R}\right) =-\angle Z$$ (49)

$$\left\{\begin{array}{rcl} \mbox{Impedance} &=& \mbox{Resistance} ... ...x{Susceptance} \\ \mbox{Admittance}&=&1/\mbox{Impedance}\\ \end{array}\right.$$ (50)

Impedance $Z$ and admittance $Y=1/Z$ are both complex variables. The real parts $Re[Z]=R$ and $Re[Y]=G$ are always positive, but the imaginary parts $Im[Z]=X$ and $Im[Y]=B$ can be either positive or negative. Therefore $Z$ and $Y$ can only be in the 1st or the 4th quadrants of the complex plane.

In particular, the admittances of the three types of elements R, L and C are

$$Y_R=\frac{1}{R},\;\;\;\; Y_L=\frac{1}{Z_L}=\frac{1}{j\omega L}=\frac{-j}{\omega L},\;\;\;\; Y_C=\frac{1}{Z_C}=\frac{1}{1/j\omega C}=j\omega C$$ (51)

Ohm's law can also be expressed in terms of admittance as well as impedance. Sometimes it is more convenient in circuit analysis to use admittance instead of impedance.

• Components parallel:

  $$Z_{total}=\frac{Z_1\;Z_2}{Z_1+Z_2},\;\;\;\;Y_{total}=Y_1+Y_2$$ (52)

• Components in series:

  $$Z_{total}=Z_1+Z_2,\;\;\;\;Y_{total}=\frac{Y_1\;Y_2}{Y_1+Y_2}$$ (53)

Generalized Ohm's law and Kirchhoff's Laws

In general, all methods such as Ohm's law and Kirchhoff's Laws used for DC circuits composed of resistors can be generalized to AC circuits composed of capacitors, inductors, as well as resistors, all represented by their impedances. Also, if we assume all voltages and currents in a circuit are sinusoids of same frequency $\omega$, they can be represented as complex phasors.

The Ohm's law can be generalized to become:

$$Z=\frac{\dot{V}}{\dot{I}},\;\;\;\;\;\;\dot{I}=\frac{\dot{V}}{Z}, \;\;\;\;\;\dot{V}=Z\dot{I}$$ (54)

and the Kirchhoff's laws can now be stated as:

• Current Law (KCL): The vector sum of the currents into a node is zero $\sum_k \dot{I}_k=0$.
• Voltage Law (KVL): The vector sum of the voltages around a loop is zero $\sum_k \dot{V}_k=0$.

Solving AC circuit by phasor method

If only the steady state solutions of the DE describing an AC circuit is of interest, the phasor method can be used to solve the problem algebraically without solving the DEs. Specifically, all sinusoidal variables are represented as phasors in terms of their amplitudes and phases, and all components in the circuit (L and C, as well as R) are represented by their impedances, so that all the laws (Ohm's law, KCL and KVL, current and voltage dividers, parallel and series combinations of components) and methods (loop current and node voltage methods, Thevenin's and Norton's theorems, etc.) discussed for DC circuit can be applied.

Operations on sinusoidal variables based on the trigonometric identities are in general lengthy and tedious. The phasor method can convert such sinusoidal variables to vectors in complex plane and thereby simplify the operations.

Here is a review of complex arithmetic.

Example 1:

Solve the circuit below. The voltage from the generator is $v(t)=28.3\;\cos(5000t+45^\circ)\;V=20\sqrt{2}\;\cos(5000t+45^\circ)$.

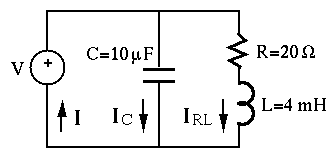
The given voltage $v(t)\;V$ can be expressed in phasor form as

$$\dot{V}=28.3 e^{j\,45 \circ} =28.3\;\angle{45^\circ}=20\sqrt{2}\;\angle{45^\circ} =20+j\;20$$ (55)

First find the impedances and admittances of the components and the two branches. As $\omega=5000$, we get

• $Y_C=j\omega C=j\;5000\times 10^{-5}=j0.05=0.05\angle{90^\circ}$

  $Z_C=1/Y_C=1/j0.05=-j20=20\angle{-90^\circ}$

• $Z_L=j\omega L=j5000\times 4\times 10^{-3}=0+j20=20\angle{90^\circ}$

  $Y_L=1/Z_L=-j0.05=0.05\angle{-90^\circ}$

• $Z_R=R=20+j0=20\angle{0^\circ}$

• $Z_{RL}=Z_R+Z_L=20+j20=20\sqrt{2}\angle{45^\circ}$ $Y_{RL}=1/Z_{RL}=1/20\sqrt{2}\angle{45^\circ} =0.025\sqrt{2}\angle{-45^\circ}=0.025-j0.025$

• $Y_{load}=Y_C+Y_{RL}=0.025+j0.025=0.025\sqrt{2}\angle{45^\circ}$

Next find all currents in phasor form:

• $\dot{I}_{load}=Y_{load}\dot{V} =(0.025\sqrt{2}\angle{45^\circ})\;(28.3\angle{45^\circ}) =1\,\angle{90^\circ}=j$

  $i_{load}(t)=Re[\dot{I} e^{j5000t}]=Re[(1\,e^{j\,90^\circ}) e^{j5000t}] =\cos(5000t+90^\circ)$

• $\dot{I}_C=Y_C\dot{V}=(0.05\angle{90^\circ})(28.3\angle{45^\circ}) =1.41\angle{135^\circ}=-1+j$

  $i_C(t)=Re[ (1.41 e^{j 135^\circ}) e^{j5000t}]=1.41\;\cos(5000t+135^\circ)$

• $\dot{I}_{RL}=Y_{RL}\dot{V} =(0.025\sqrt{2}\angle{-45^\circ})(28.3\angle{45^\circ})=1$

  $i_{RL}(t)=Re[ e^{j0} e^{j5000t} ]=\cos(5000t)$

• $\dot{V}_R=Z_R\dot{I}_{RL}=(20\angle{0^\circ})(1\;\angle{0^\circ}) =20\;\angle{0^\circ}=20+j0$

  $v_R(t)=20\;\cos(5000t)$

• $\dot{V}_L=Z_L\dot{I}_{RL}=(20\angle{90^\circ})(1\;\angle{0^\circ}) =20\;\angle{90^\circ}=0+j20$

  $v_L(t)=20\;\cos(5000t+90^\circ)$

Verify:

• $\dot{V}_R+\dot{V}_L=10\sqrt{2}+j10\sqrt{2}=20\angle{45^\circ} =\dot{V}$
• $\dot{I}_C+\dot{I}_{RL}=0.5\sqrt{2}-0.5\sqrt{2}+j0.5\sqrt{2} =0.5\sqrt{2}\angle{90^\circ}=\dot{I}_{load}$

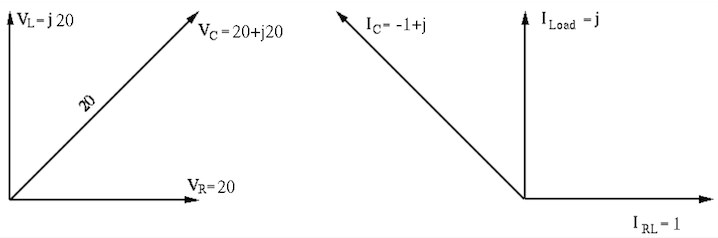
Example 2:

A current $i(t)=17\;\cos(1000t+90^\circ)=12\sqrt{2}\cos(1000t+90^\circ)$ flows through a circuit composed of a resistor $R=18\Omega$, a capacitor $C=83.3\mu F=83.3\times 10^{-6}F$, and an inductor $L=30 mH=30\times 10^{-3}H$ connected in series. Find the resulting voltage across all three elements.

• Express $i(t)$ in phasor: $\dot{I}=12\sqrt{2}\;\angle{90^\circ} =j\;12\sqrt{2}$.
• Find impedance for each element ( $\omega=1000$):

  $$Z_R=R=18,\;\;\;\;Z_C=1/j\omega C=12\angle{-90^\circ}=0-j12,\;\;\;\; Z_L=j\omega L=30\angle{90^\circ}=0+j30$$ (56)

• Find overall impedance:

  $$Z_{total}=Z_R+Z_C+Z_L=18+j(-12+30)=18+j18=18\sqrt{2}\angle{45^\circ}$$ (57)

• Find voltage across all three elements:

  $$\dot{V}_{total}=\dot{I}Z_{total}=(12\sqrt{2}\;\angle{90^\circ})\;(18\sqrt{2}\angle{45^\circ}) =432\;\angle{135^\circ}$$ (58)

  $$v(t)=432\;\cos(1000t+135^\circ)$$ (59)

Alternatively, we can find voltage across each of the elements:

$$\dot{V}_R = \dot{I}Z_R=216\sqrt{2}\;j=305.5\;\angle{90^\circ}$$

$$\dot{V}_C = \dot{I}Z_C=144\sqrt{2}\;\angle{0^\circ}=203.6$$

$$\dot{V}_L = \dot{I}Z_L=360\sqrt{2}\;\angle{180^\circ}=510\;\angle{180^\circ}$$ (60)

or in time domain:

$$v_R(t) = 305.5\; \cos(1000t+\pi/2)$$

$$v_C(t) = 203.6\; \cos(1000t)$$

$$v_L(t) = 509\; \cos(1000t+\pi)$$ (61)

Adding $\dot{V}_R$, $\dot{V}_C$, and $\dot{V}_L$, we get the total voltage which is the same as what we got above:

$$\dot{V}_{total}=\dot{V}_R+\dot{V}_C+\dot{V}_L =216\sqrt{2}\angle{90^\circ}+216\sqrt{2}\angle{180^\circ}=432 \angle{135^\circ}$$ (62)

Example 3:

In the circuit below, $v_S(t)=A\cos(\omega t)=A\cos(10^6 t)$ with some unknown peak value $A$, $R_1=R_2=R_3=R=10\Omega$, and $L=10\,\mu H$. The RMS value of $v_2$ across $R_2$ is 10 V. It is also known that $v_s(t)$ and $i_s(t)$ are in phase.

• Find $C$.
• Find the RMS values of $v_C(t)$ and $v_L(t)$.
• Find the RMS values of $v_{cd}=v_{RC}=V_{RL}$ and $v_{ab}$.
• Find the peak value $A$ of $v_s(t)$.

Solution

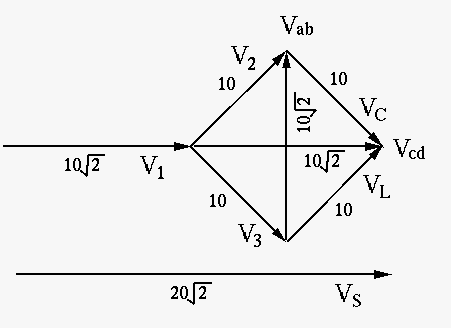
We first note that $\dot{V}_C$ is behind $\dot{V}_2=\dot{I}_2R_2$ by $\pi/2$, and $\dot{V}_L$ is ahead of $\dot{V}_3=\dot{I}_3R_3$ by $\pi/2$ (“ELI the ICE man”). Also, as $v_s(t)$ and $i_s(t)$ are in phase, the parallel combination of the RL and RC branches introduces no phase shift, i.e., its impedance shown below must be real:

$$Z_{RL}(\omega)\vert\vert Z_{RC}(\omega)=\frac{(R+jX_L)(R+jX_C)}{R+jX_L+R+jX_C} =\frac{R^2-X_LX_C+j(X_L+X_C)}{2R+j(X_L+X_C)}$$ (63)

i.e., $X_C+X_L=0$. We therefore get $1/j\omega C=-j\omega L=-j 10$ and $C=10^{-7}=0.1\mu F$, $Z_{RL}=10+j\,10$, $Z_{RC}=10-j\,10$, $\vert Z_{RL}\vert=\vert Z_{RC}\vert=\sqrt{10^2+10^2}=10\sqrt{2}$, i.e.,

$$Z_{RL}(\omega)\vert\vert Z_{RC}(\omega)=\frac{(10+j10)(10-j10)}{10+j10+10-j10}=10$$ (64)

As $\vert R_2\vert=\vert Z_C\vert=10 \Omega$, $\vert\dot{V}_C\vert=\vert\dot{V}_R\vert=10V$. But as they are $\pi/2$ apart in phase, we have $\vert\dot{V}_{cd}\vert=\vert\dot{V}_{RC}\vert=\vert\dot{V}_{RL}\vert=10\sqrt{2}$, and from the vector diagram $\vert\dot{V}_{ab}\vert=10\sqrt{2}$. We also get the currents through RC and RL branches are:

$$\vert\dot{I}_{RC}\vert=\frac{\vert\dot{V}_{RC}\vert}{\vert Z_{RC}... ...t{I}_{RL}\vert=\frac{\vert\dot{V}_{RL}\vert}{\vert Z_{RL}\vert}=\frac{10}{10}=1$$ (65)

But their phase difference is $\pi/2$, we have

$$\vert\dot{I}_s\vert=\vert\dot{I}_{RL}+\dot{I}_{RC}\vert=\sqrt{2}$$ (66)

The voltage across $R_1$ is $V_1=RI_s=10\sqrt{2}$, and

$$\dot{V}_s=\dot{V}_1+\dot{V}_{cd}=10\sqrt{2}+10\sqrt{2}=20\sqrt{2}$$ (67)

The peak value is therefore $A=\sqrt{2} V_s=40\,V$