Next: Butterworth filters Up: Chapter 6: Active Filter Previous: The Twin-T notch (band-stop)
The Wien bridge is a particular type of the Wheatstone bridge of which two of the four arms are composed of a capacitor as well as a resistor in parallel and series:

For this bridge to balance, the ratios of the left and right branches should be the same:
 (50)
(50)
 (51)
(51)
 (52)
(52)
 and
and  , we have:
, we have:
 (53)
(53)
 (54)
(54)
Wien-Robinson Filter
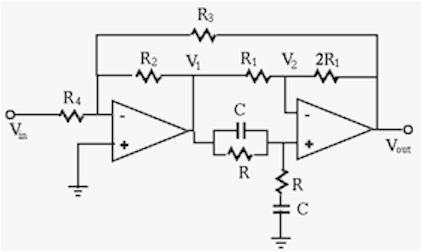
 (55)
(55)
 (56)
(56)
 , i.e.,
, i.e.,
 (57)
(57)
 (58)
(58)
 (59)
(59)
 (60)
(60)
 (61)
(61)
 (62)
(62)
 (63)
(63)
 (64)
(64)
 (65)
(65)
 (66)
(66)
 ,
stop-band
,
stop-band
 and
and
 .
.
Further reading for