Colpitts Oscillators

Oscillation in a circuit is undesirable if the circuit is an amplifier or part of a control system which needs to be stable without oscillation. However, oscillation is desirable in many applications such as sinusoidal signal generator, carrier signal generation is broadcast transmission (radio and TV), clock signal in digital systems, etc.

An oscillator is a feedback system composed of a forward path with gain $G(j\omega)$ and a feedback path with gain $F(j\omega)$:

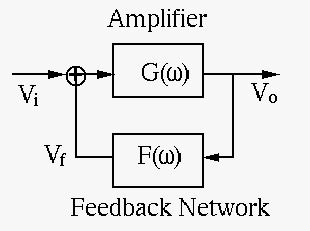
For the system to oscillate at a certain frequency, the feedback needs to be positive for the frequency to be positively reinforced while passing through the forward path in order to sustain the output $V_o$ with zero input $V_i=0$. Specifically, the output $V_o$ and the input $V_i$ of a feedback system are related by

$$V_o=G(V_i+FV_o)=GV_i+GFV_o,\;\;\;\;\;\;\;\;\;\frac{V_o}{V_i}=H=\frac{G}{1-GF}, \;\;\;\;\;\;\;V_o=HV_i=\frac{G}{1-GF}\,V_i$$ (142)

where $GF$ is the open-loop gain and $H$ is the closed-loop gain. For this system to oscillate, i.e., for it to produce an output with zero input, its closed-loop gain needs to be infinite, i.e., its open-loop gain $GF$ need to be real, with zero phase $\angle(GF)=0$ and unit gain $\vert GF\vert=1$.

There exist many different configurations of oscillators based on a single transistor. Shown below are three typical Colpitts oscillators: common-base (CB, left), common emitter (CE, middle), and common collector (CC, right). All such circuits contain a “tank” LC circuit composed of an inductor $L$ in parallel with $C_1$ and $C_2$ in series, with a resonant frequency

$$\omega_0=\frac{1}{\sqrt{LC_s}},\;\;\;\;\;\; \;\;\;\;\; C_s=\left(\frac{1}{C_1}+\frac{1}{C_2}\right)^{-1}$$ (143)

where $C_s$ is the equivalent capacitance of the series combination of $C_1$ and $C_2$. All other $C$s (without a subscript) are coupling capacitors that have a large enough capacitance and can therefore be treated as short circuit for AC signals.

Here are the requirements for these circuits to oscillate:

1. an LC tank tuning circuit that generates sinusoidal oscillation at its resonant frequency $\omega_0=1/\sqrt{LC_s}$
2. a positive feedback loop that sustains the oscillation.

How each of these circuits works can be qualitatively understood as below:

• CB with the base AC grounded: The collector voltage $v_c$ is the output, a fraction of which at the middle point between the two capacitors, “tap point”, is fed-back to the emitter to a positive feedback loop:

  $$v_c\uparrow\Longrightarrow v_e\uparrow\Longrightarrow v_{be}\down... ...b\downarrow \Longrightarrow i_c=\beta i_b\downarrow \Longrightarrow v_c\uparrow$$ (144)

• CE with the emitter AC grounded: The collector voltage $v_c$ is the output, which is fed-back through the LC tank circuit to the base. As the tap point is grounded, the sinusoidal voltage across the LC tank produces opposite voltage polarities at the far ends of $C_1$ and $C_2$, i.e., $v_{C_1}=v_b$ and $v_{C_2}=v_c$ have opposite phases and thereby form a positive feedback loop:

  $$v_c\uparrow\Longrightarrow v_b\downarrow\Longrightarrow v_{be}\do... ...b\downarrow \Longrightarrow i_c=\beta i_b\downarrow \Longrightarrow v_c\uparrow$$ (145)

• CC with the collector AC grounded: This a voltage follower circuit in which the emitter voltage $v_e$ is the output that follows the input voltage $v_b$. The feedback from the emitter through the LC tank circuit to the base form a positive feedback loop:

  $$v_e=v_t\uparrow\Longrightarrow v_b\uparrow \Longrightarrow i_b\uparrow\Longrightarrow i_e=(\beta+1)i_b\uparrow \Longrightarrow v_e\uparrow$$ (146)

  where $v_t$ is the voltage at the tap point.

More specifically, we consider the common-collector circuit as an example. To find out why the circuit oscillates and the resonant frequency, we disconnect the base path of the circuit and consider the open-loop gain of $V_o/V_i$ of the feedback loop. We further model the transistor by a Thevenin voltage source $V_i$ in series with an internal $R$, as shown in the figure:

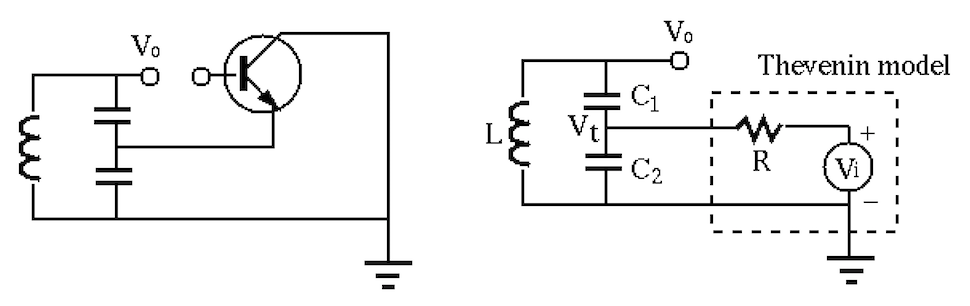
As the load of the Thevenin source, the tank circuit receives an input $V_t$ at the tap point, and produces an output $V_o$ across the parallel combination of $L$ and $C_1$ in series with $C_2$. Applying KCL at the tap point we get:

$$\frac{V_t-V_i}{R}+\frac{V_t}{1/j\omega C_2}+\frac{V_t}{j\omega L+1/j\omega C_1}=0$$ (147)

i.e.,

$$V_t\left(\frac{1}{R}+j\omega C_2+\frac{j\omega C_1}{1-\omega^2LC_1}\right) =\frac{V_i}{R}$$ (148)

Solving for $V_t$ we get

$$V_t=\frac{1}{R(\frac{1}{R}+j\omega C_2+\frac{j\omega C_1} {1-\omega^2LC_1})}\;V_i =\frac{1}{1+j\omega R(C_2+C_1/(1-\omega^2LC_1)}\;V_i$$ (149)

which is maximized if the frequency is such that the imaginary part of the denominator is zero:

$$C_2+\frac{C_1}{1-\omega_0^2LC_1}=0,\;\;\;\;\;\;\; \;\;\;\;\; \omega_0=\frac{1}{\sqrt{LC_1C_2/(C_1+C_2)}}=\frac{1}{\sqrt{LC_s}}$$ (150)

Here $\omega_0$ is the resonant frequency, at which the voltage $V_t$ become the same as the source voltage $V_t=V_i$, as the impedance of the tank circuit as the load of the Thevenin source is infinity:

$$Z_{tank} = Z_{C_2}\vert\vert(Z_{C_1}+Z_L)=\frac{Z_{C_2}(Z_{C_2}+Z_L)}{Z_{C_2... ...{(1/j\omega C_1+j\omega L)/j\omega C_2} {1/j\omega C_2+1/j\omega C_1+j\omega L}$$

$$= \frac{(1/j\omega C_1+j\omega L)/C_2}{1/C_1+1/C_2-\omega^2 L} =\fr... ..._2}{1/C_s-\omega^2 L} \;\;\stackrel{\omega=\omega_0}{\Longrightarrow}\;\;\infty$$ (151)

When $\omega=\omega_0$, the denominator becomes zeros and $Z_{tank}=\infty$, i.e., there is no current drawn from the source by the tank circuit. Consequently, the voltage drop across $R$ is zero and the voltage received by the tank circuit is $V_t=V_i$. Now the output voltage $V_o$ can be found by voltage divider:

$$V_t=\frac{Z_{C_2}}{Z_{C_1}+Z_{C_2}}\;V_o=\frac{C_1}{C_1+C_2}\,V_o, \;\;\;\;\; \;\;\;\;\;\;\;\; V_o=\frac{C_1+C_2}{C_1}\;V_t=\frac{C_1+C_2}{C_1}\;V_i$$ (152)

The open-loop gain (from $V_i$ to $V_o$) is:

$$\frac{V_o}{V_i}=\frac{C_1+C_2}{C_1}$$ (153)

We see that when $\omega=\omega_0$, the open-loop gain is real but greater than 1. However, the non-linearity of the transistor as the feedback path (from $V_o$ to $V_i$) will force the open-loop to be 1. The circuit is an oscillator with frequency at $\omega_0=1/\sqrt{LC_s}$.