Next: Analysis of Op-Amp Circuits Up: Chapter 5: Operational Amplifiers Previous: Chapter 5: Operational Amplifiers
The circuit schematic of the typical 741 op-amp is shown below:
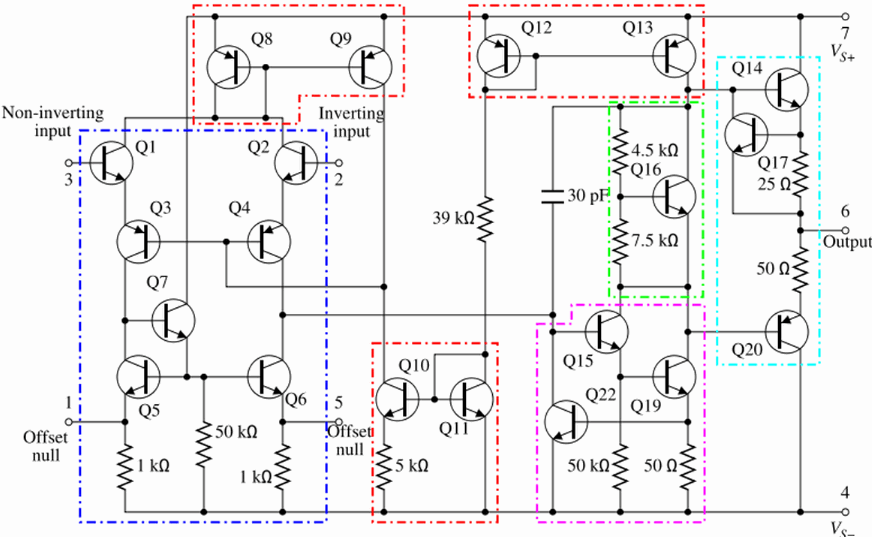
A component-level diagram of the common 741 op-amp. Dotted lines outline:
Like all op-amps, the circuit basically consists of three stages:
 .
.
 of both polarities
(typically
of both polarities
(typically  V).
V).
Although the op-amp circuit may look complicated, the analysis of its operation and behaviors can be simplfied based on the following assumptions:
 can be treated as infinity
can be treated as infinity
 .
.
 ),
and could be approximated to be zero
),
and could be approximated to be zero  .
.
 can be treated as zero
can be treated as zero
 ,
i.e., the output
,
i.e., the output  is not affected by the load
is not affected by the load  (so long as it is
much greater than
(so long as it is
much greater than  ).
).
 ), i.e., the property of the op-amp
remain unchanged for all frequencies of interest.
), i.e., the property of the op-amp
remain unchanged for all frequencies of interest.
Based on these approximations, an op-amp can be modeled in terms of the following three parameters:
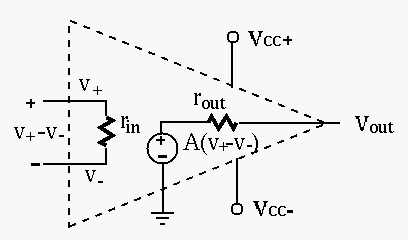
 : very large, typically a few mega-Ohms or
higher (
: very large, typically a few mega-Ohms or
higher (
 , e.g., 741
, e.g., 741
 ), depending on
the frequency and specific components used (e.g., BJT or FET).
), depending on
the frequency and specific components used (e.g., BJT or FET).
 :, very small, typically a few tens of
ohms, e.g., 75
:, very small, typically a few tens of
ohms, e.g., 75  .
.
 :, based on both the inverting input
:, based on both the inverting input  and the non-inverting input
and the non-inverting input  :
:
 |
(1) |
 is the differential-mode gain and
is the differential-mode gain and  is the common-mode gain.
It is desired that
is the common-mode gain.
It is desired that
 and
and
 , i.e., the
output is only proportional to the difference
, i.e., the
output is only proportional to the difference  between the two inputs.
The common-mode rejection ratio (CMRR) is defined as the ratio between
differential-mode gain and common-mode gain:
between the two inputs.
The common-mode rejection ratio (CMRR) is defined as the ratio between
differential-mode gain and common-mode gain:
 |
(2) |
Also, as the output








As 









 , output impedance
, output impedance
 and voltage gain
and voltage gain  , as shown in (B). Then the voltage follower
can be modeled by its input impedance
, as shown in (B). Then the voltage follower
can be modeled by its input impedance  , output impedance
, output impedance  ,
and voltage gain
,
and voltage gain  , as shown in (C).
, as shown in (C).

Specifically, 



 : Applying KVL to the loop we get
: Applying KVL to the loop we get
 |
(3) |
 we get the input impedance:
we get the input impedance:
 |
(4) |
 : The open-circuit output
voltage is
: The open-circuit output
voltage is
 |
(5) |
 |
(6) |
 and
and  . We therefore have
. We therefore have
 |
(7) |
 :
With a short-circuit load, we have
:
With a short-circuit load, we have
 , and the
short-circuit current can be found by superposition:
, and the
short-circuit current can be found by superposition:
 |
(8) |
 , we get the output impedance
(Thevenin's model):
, we get the output impedance
(Thevenin's model):
 |
(9) |

In summary, we see that the voltage follower has a unit voltage gain, but
much increased input resistance






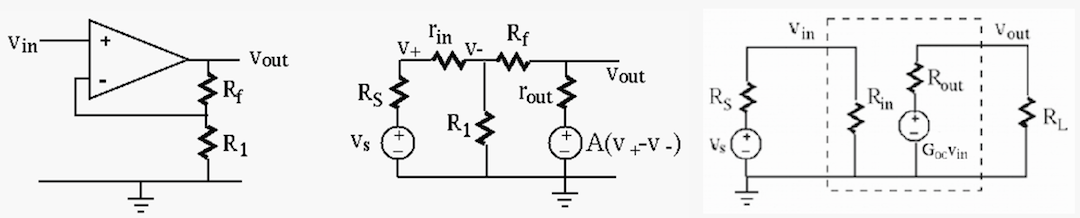
Example:

The figure on the left shows a circuit represented by an ideal voltage
source 


 |
(10) |
 is only a fraction
of the voltage due to the voltage drop
is only a fraction
of the voltage due to the voltage drop
 across the
internal resistance
across the
internal resistance  . If it is desired for the output voltage to
be as close to the source as possible, the internal resistance
. If it is desired for the output voltage to
be as close to the source as possible, the internal resistance  needs to be small compared to the load resistance
needs to be small compared to the load resistance  .
.
Next consider inserting a voltage follower (buffer), in between the
source and the load, as shown in the middle figure. The follower is
modeled by its input and output resistances 


 |
(11) |
 can be very close to
source voltage, i.e.,
can be very close to
source voltage, i.e.,
 , due to the nature of the
op-amp:
, due to the nature of the
op-amp:




As the analysis of the circuit using full model of the op-amp is very involved, certain approximation is made to simplify the analysis.
 :
:
As







 |
(12) |
 we get
we get
 |
(13) |
![$\displaystyle v_{out}=v_s-(R_1+R_f) i_{in}
=v_s\left[1-\frac{(A+1)(R_1+R_f)}{(A+1)R_1+R_f}\right]
=v_s\frac{-AR_f}{(A+1)R_1+R_f}$](img75.svg) |
(14) |
 |
(15) |
 .
.
We assume 




 |
(16) |
 :
:
 |
(17) |
 |
 |
![$\displaystyle \frac{v_s-v^-}{R_1}
=\frac{v_s}{R_1}\left[1-\frac{R_f}{R_f+(A+1)R_1}\right]$](img83.svg) |
|
 |
 |
(18) |
 by
by  we get
we get
 |
(19) |
 !
!
 to simplify the analysis.
to simplify the analysis.
 |
(20) |
 , the above becomes
, the above becomes
 |
(21) |
 we get
we get
 |
(22) |
 we get
we get
 |
(23) |
 |
 |
![$\displaystyle [v^--(-Av^-)]\frac{r_{out}}{R_f+r_{out}}-Av^-
=v^- \left(\frac{r_{out}-AR_f}{R_f+r_{out}}\right)$](img92.svg) |
|
 |
 |
(24) |
 |
 |
 |
|
 |
 |
(25) |
 and
and
 .
.
In summary,
 |
(26) |
 |
(27) |
 |
(28) |
The three parameters of this non-inverting amplifier can be found to be (see here):
 |
(29) |
 |
(30) |
 |
(31) |
 is a little better, but both
is a little better, but both  and
and  are
a little worse. In particular if
are
a little worse. In particular if  , this non-inverting amplifier
becomes a voltage follower with
, this non-inverting amplifier
becomes a voltage follower with
 ,
,
 ,
and
,
and
 .
.