First and Second Order Low/High/Band-Pass filters

Consider the two active filters shown below and determine what kind of filters they are.

Low-pass filter:

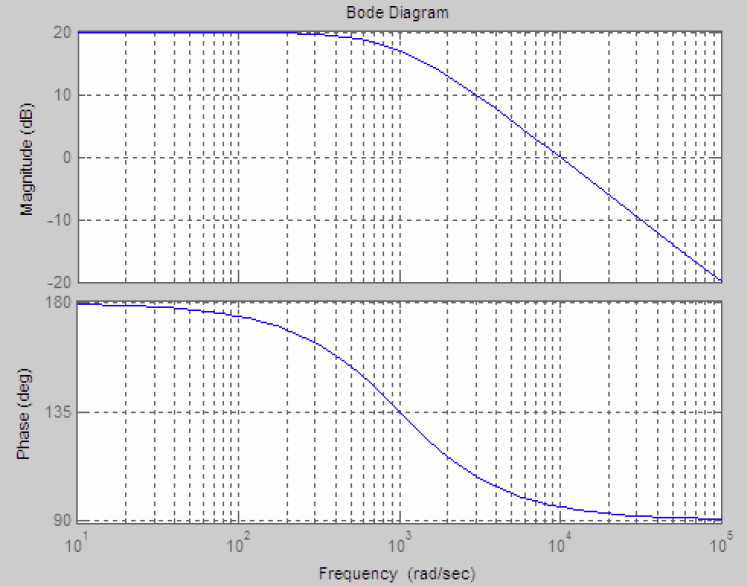
Qualitatively, we see that this is a low-pass filter as

$\displaystyle H(j\omega)=\frac{V_{out}}{V_{in}}=\left\{\begin{array}{ll} -R_2/R_1 & \omega=0\\ 0 & \omega\rightarrow\infty \end{array}\right.$ (1)

The second case is due to the fact that $Z_C=1/j\omega C\rightarrow 0$ when $\omega\rightarrow\infty$ .

Specifically,

$\displaystyle H(j\omega)$	$\textstyle =$	$\displaystyle -\frac{Z_2}{Z_1}=-\frac{R_2\;\vert\vert\;(1/j\omega C)}{R_1} =-\f... ...\frac{R_2/j\omega C}{R_2+1/j\omega C} =-\frac{R_2}{R_1}\frac{1}{j\omega R_2C+1}$
	$\textstyle =$	$\displaystyle -H(0)\frac{1}{1+j\omega \tau}$	(2)

where

is the DC gain when $\omega=0$ , and $\omega_c=1/\tau=1/R_2C$ is cut-off or corner frequency, at which $\vert H(j\omega_c)\vert=H(0)/\sqrt{2}$ . Intuitively, when frequency is high, $Z_2(j\omega)$ is small and the negative feedback becomes strong, and the output is low. For example, when $\tau=10^{-3}$ , $\omega_c=1/\tau=10^3$ , the Bode plots are shown below:

High-pass filter:

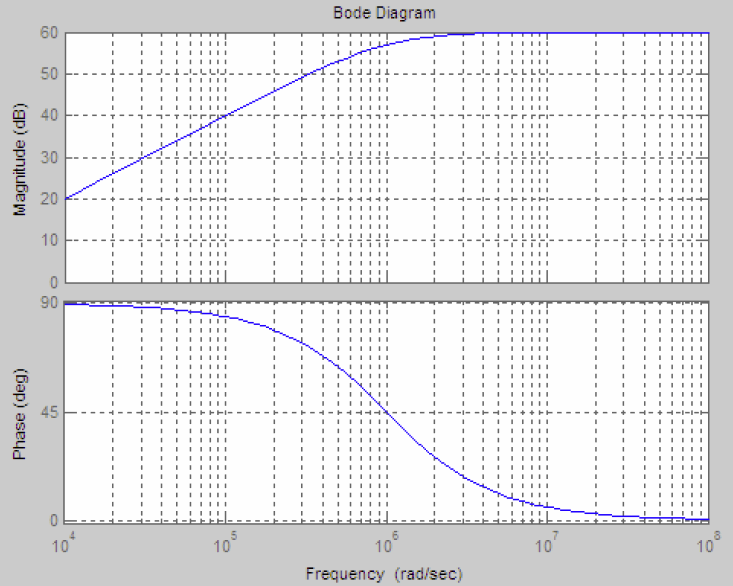
Qualitatively, we see that this is a high-pass filter as

$\displaystyle H(j\omega)=\frac{V_{out}}{V_{in}}=\left\{\begin{array}{ll} 0 & \omega\rightarrow 0\\ -R_2/R_1 & \omega\rightarrow\infty \end{array}\right.$ (3)

The first case is due to the fact that $Z_C=1/j\omega C\rightarrow\infty$ when $\omega=0$ .

Specifically,

$\displaystyle H(j\omega)$	$\textstyle =$	$\displaystyle -\frac{Z_2(j\omega)}{Z_1(j\omega)} =-\frac{R_2}{R_1+1/j\omega C} =-\frac{R_2}{R_1}\;\frac{1}{1+1/j\omega R_1C}$
	$\textstyle =$	$\displaystyle -H(\infty)\;\frac{1}{1+1/j\omega\tau}$	(4)

where $H(\infty)=R_2/R_1$ is the gain when $\omega\rightarrow\infty$ , $\omega_c=1/\tau=1/R_1C$ is the cut-off or corner frequency, at which $\vert H(j\omega_c)\vert=H(\infty)/\sqrt{2}$ . Intuitively, when frequency is low $Z_1(j\omega)$ is large and the signal is difficult to pass, therefore the output is low. For example, when $\tau=10^{-6}$ , $\omega_c=1/\tau=10^6$ , the Bode plots are shown below:

If we let $R_1=R_2$ , i.e., $H(0)=1$ , and ignore the negative sign ( $180^\circ$ phase shift), the low-pass and high-pass filters can be represented by their transfer functions with $s=j\omega$ :

$\displaystyle H_{lp}(j\omega)=\frac{\omega_c}{j\omega+\omega_c} =\frac{1}{j\om... ...\omega)=\frac{j\omega}{j\omega+\omega_c} =\frac{\j\omega\tau}{j\omega\tau+1}$ (5)

Second Order Band-pass Filters:

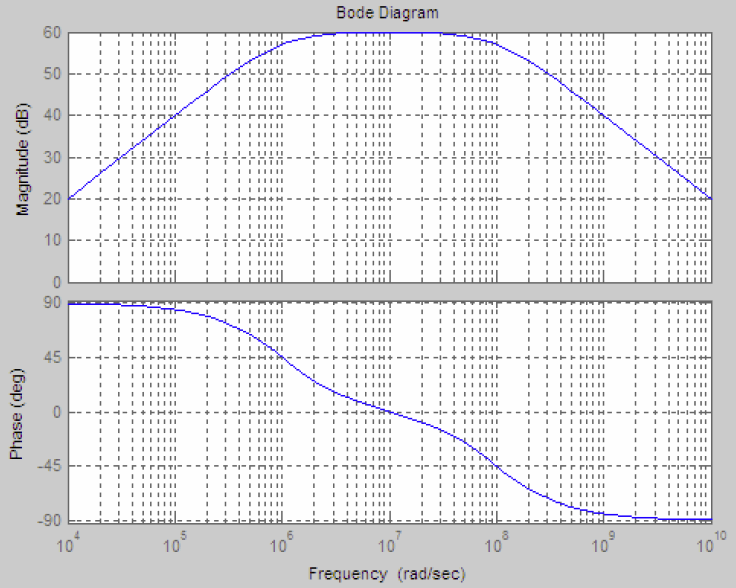
Qualitatively, we see that this is a band-pass filter as

$\displaystyle H(j\omega)=\frac{V_{out}}{V_{in}}=\left\{\begin{array}{ll} 0 & \omega=0 \\ 0 & \omega\rightarrow\infty \end{array}\right.$ (6)

The second case is due to the fact that $Z_C=1/j\omega C\rightarrow 0$ when $\omega\rightarrow\infty$ .

We let

$\displaystyle Z_1(\omega)=R_1+(1/j\omega C_1),\;\;\;\;\;\;\; Z_2(\omega)=R_2\vert\vert(1/j\omega C_2)$ (7)

and get the FRF of this inverting amplifier as

$\displaystyle H_{BP}(j\omega)$	$\textstyle =$	$\displaystyle -\frac{Z_2(\omega)}{Z_1(\omega)} =-\frac{R_2\vert\vert(1/j\omega ... ...1/j\omega C_1} =-\frac{R_2/j\omega C_2}{(R_1+1/j\omega C_1)(R_2+1/j\omega C_2)}$
	$\textstyle =$	$\displaystyle -\frac{j\omega R_2C_1}{(j\omega R_1C_1+1)(j\omega C_2R_2+1)} =-\frac{j\omega \tau_3}{(1+j\omega \tau_1)(1+j\omega \tau_2)}$
	$\textstyle =$	$\displaystyle -\left(j\omega\tau_3\right)\;\left(\frac{1}{1+j\omega\tau_1}\right) \;\left(\frac{1}{1+j\omega\tau_2}\right)$
	$\textstyle =$	$\displaystyle -\left(\frac{\tau_3/\tau_1\tau_2\;j\omega} {(j\omega)^2+j\omega(\... ...\omega} \; \frac{j\omega\Delta\omega}{-\omega^2+j\omega\Delta\omega+\omega_n^2}$
	$\textstyle =$	$\displaystyle -\frac{\tau_3}{\tau_1+\tau_2}\; \frac{j\omega\Delta\omega}{-\omega^2+j\omega\Delta\omega+\omega_n^2}$	(8)

where $\tau_1=R_1C_1$ , $\tau_2=R_2C_2$ , $\tau_3=R_2C_1$ , $\Delta\omega=(\tau_1+\tau_2)/\tau_1\tau_2$ , and $\omega_n=1/\sqrt{\tau_1\tau_2}$ . This is a bandpass filter with the peak at the natural frequency $\omega_n$ , which is the geometric average of the two corner frequencies $\omega_1=1/\tau_1$ and $\omega_2=1/\tau_2$ , or the arithmetic average of $\omega_1$ and $\omega_2$ in log scale:

$\displaystyle \omega_n=1/\sqrt{\tau_1\tau_2}=\sqrt{\omega_1\omega_2}, \;\;\;\;... ...r}\;\;\;\;\; \log\omega_n=\frac{1}{2}\left(\log\omega_1+\log\omega_2\right)$ (9)

For example, if $\omega_1=1/\tau_1=10^6$ , $\omega_2=1/\tau_2=10^8$ , $\omega_3=1/\tau_3=10^3$ , then $\omega_n=\sqrt{\omega_1\omega_2}=10^7$ . When $\omega=\omega_n$ , we have

$\displaystyle LM(\omega_n)=20\log\vert H(\omega_n)\vert=20\log\left(\frac{\tau_... ... =20\log\left(\frac{10^{-3}}{10^{-6}+10^{-8}}\right) \approx 20\log 10^3=60$ (10)

The Bode plots are shown below:

Second Order Band-stop Filters:

If the log-magnitude $Lm$ of the Bode plot of a band-pass filter is vertically flipped, we get a band-stop filter:

$\displaystyle -Lm_{BP}(j\omega)=-20\log \vert H_{BP}(j\omega)\vert =-20\log \b... ...\log \bigg\vert \frac{Z_1(\omega)}{Z_2(\omega)} \bigg\vert =Lm_{BS}(j\omega)$ (11)

We therefore see that if we simply swap $Z_1(\omega)$ and $Z_2(\omega)$ in the op-ammp circuit of the band-pass filter, we get a band-stop filter:

$\displaystyle H_{BS}(j\omega)$	$\textstyle =$	$\displaystyle -\frac{Z_1(\omega)}{Z_2(\omega)} =-\frac{R_2+1/j\omega C_2}{R_1\v... ... 1/j\omega C_1} =-\frac{R_2+1/j\omega C_2}{R_1/j\omega C_1/(R_1+1/j\omega C_1)}$
	$\textstyle =$	$\displaystyle \frac{(1+j\omega C_1R_1)(1+j\omega C_2R_2)}{j\omega R_1C_2} =\frac{(1+j\omega\tau_1)(1+j\omega\tau_1)}{j\omega\tau_3}$	(12)