Theory of Color Matching

Now we can revisit the color matching issue, and discuss the real meaning of the equivalent symbol 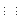$\equiv$.

The visual system and the color system are described by

• The Visual System: The three types of photoreceptors with sensitivities $S_i(\lambda),\;\;(i=1,2,3)$;
• The Color System: The three primaries with energy spectral distributions $P_j(\lambda),\;\;(j=1,2,3)$ together with the reference white $W(\lambda)$.

We want to match a given color $L(\lambda)$ with another color produced by mixing the three primaries

$$L'(\lambda)=\sum_{j=1}^3 A_j(L) P_j(\lambda)$$

with proper weights or intensities $A_j(L),\;\;(j=1,2,3)$.

Since color matching now means specifically that the three types of photoreceptors have the same responses to the two colors, we must require

$$r_i(L) = \int L(\lambda) S_i(\lambda) d\lambda$$

$$= r_i(L')= \int L'(\lambda) S_i(\lambda) d\lambda = \int [ \sum_{j=1}^3 A_j(L) P_j(\lambda) ] S_i(\lambda) d\lambda$$

$$= \sum_{j=1}^3 A_j(L) \int S_i(\lambda)P_j(\lambda)d\lambda = \sum_{j=1}^3 A_j(L) a_{ij}\;\;\;(i=1,2,3)$$

where $a_{ij}$ is defined as the response of the ith photoreceptors to the jth primary:

$$a_{ij}\stackrel{\triangle}{=}r_i(P_j)= \int S_i(\lambda)P_j(\lambda) d\lambda \;\;\;\;\;(i,j=1,2,3)$$

The three equations above can now be rewritten to obtain the color matching equations:

$$\sum_{j=1}^3 a_{ij} A_j(L) =\int S_i(\lambda) L(\lambda) d\lambda \;\;\;(i=1,2,3)$$

Solving this set of three simultaneous equations, we get the three coefficients $A_j(L),\;\;(i=1,2,3)$, the weights for mixing the three primaries to produce color $L'(\lambda)=\sum_j A_j(L) P_j(\lambda)$, which will be perceived the same as the given color $L(\lambda)$:

$$L(\lambda) \equiv L'(\lambda)=\sum_{j=1}^3 A_j(L) P_j(\lambda)$$

However, we note that these two functions do not equate mathematically in general, i.e., they are different energy spectral distributions:

$$L(\lambda) \neq L'(\lambda)=\sum_{j=1}^3 A_j(L) P_j(\lambda)$$

In general if the three types of photoreceptors' responses to two colors $L_1(\lambda)$ and $L_2(\lambda)$ are the same, i.e.,

$$r_i(L_1)=\int L_1(\lambda) S_i(\lambda) d\lambda= r_i(L_2)=\int L_2(\lambda) S_i(\lambda) d\lambda\;\;\;(i=1,2,3)$$

then the two colors are indistinguishable and are called metamers. There are infinitely many possible metameric spectral energy distributions that cause the same responses in the visual system.